Following Instructions
Drawing no.1
Draw a square and try to divide it in its most natural and simplest way, so that you can use those forms or division lines as decoration.
Sophie Taeuber-Arp

In the first instructions you are asked to draw a square and divide it. Sophie Taeuber-Arp is essentially asking you to make an ‘element’. You can understand an ‘element’ as the smallest indivisible component of a pattern. A repeat pattern can be made by using ‘elements’ only. Because you are asked to make a square in the first instance it also operates as a ‘cell’, a square is a polygon which can be fitted together without any spaces in between and be repeated in a pattern.
Sophie Taeuber-Arp sets out in the first instruction with the most basic and core of making a pattern the ‘element’. Yet she gives you options: how does one divide a square? If you divide it vertically, it tends to lead to a more vertical underlying structure, likewise different division leads to horizontal or diagonal structure. In the first instruction there is no mention of colour, so I decided just to use black gouache.
Drawing no.2
Draw a square and try to divide it in its most natural and simplest way, so that you can use those forms or division lines as decoration.
A further exercise is trying to divide it more complex and paint the different fields with two or three clear colours.
Sophie Taeuber-Arp

This instruction invites you to divide with greater complexity.
In order to do so, you need to reflect on and analyse the first pattern. What is the core structure? How can I build on this?
Due to using only black in the first instructions it was easier to discern the core structure in the pattern.
In this second instruction Sophie Taeuber-Arp also introduces colour which opens up new possibilities for applying a colour structure. One can start to play with core structure, reinforcing or dissolving the structure by degrees.
Drawing no.3
Drawing no.4
Draw a square and try to divide it in its most natural and simplest way, so that you can use those forms or division lines as decoration.
A further exercise is trying to divide it more complex and paint the different fields with two or three clear colours.
Try to do the same with a circle.
Sophie Taeuber-Arp
Sophie Taeuber-Arp introduces you to a new shape; a circle. It is a very different ‘element’ from a square and it is not a polygon. By doing so you are being encouraged to deepen your thought about the choice of ‘element’ and how it creates new consequences for pattern development. As soon as you put circles together you create a negative space between them. At this stage Sophie Taeuber-Arp does not emphasise the importance of this and leaves it until you have more experience.
Drawing no.5
Put a very small shape right next to the same very big shape and repeat this several times next to each other.
Try to achieve a good rhythm from this, for example by putting three small shapes next to a big one or three forms of decreasing size several times after each other. Make sure when using simple shape that the negative one is always as good as the positive. We call a negative shape the room between two forms creating the ornaments.
Sophie Taeuber-Arp

By putting a small shape right next to the same very big form you are making a ‘motif’, used to create a repeat pattern.
Sophie Taeuber-Arp invites you to repeat this several times but leaves it open for you to decide which direction to repeat. You are essentially making a linear symmetry, which leaves options for creating a repeat pattern.
Intuitively one starts to try out possibilities. It is also somewhat left open for you to embrace what are seen as the four basic operations for making pattern: Reflection, Rotation, Translation and Glide Reflection.
Drawing no.6
Put a very small shape right next to the same very big shape and repeat this several times next to each other.
Try to achieve a good rhythm from this, for example by putting three small shapes next to a big one or three forms of decreasing size several times after each other. Make sure when using simple shapes that the negative one is always as good as the positive. We call a negative space the room between two shapes creating the ornaments.
Sophie Taeuber-Arp

By pointing out that the importance of shapes and their negative spaces are equally important, you are made aware of the distance between the shapes when making a repeat pattern. There are many variations of a pattern you can make by just experimenting with spacing.
Drawing no.7
You could also start with the line. Try to see what expression you could achieve with different wavy lines or jagged lines.
Try to interweave those lines in complicated ways.
Sophie Taeuber-Arp

In the instruction no.6 you were invited to consider spacing carefully and intuitively. Now you are starting to play with spacing of wavy or zigzag lines.
There is no guidance on colour and it is up to you decide if is a pattern consisting of line only or if you want to use colour.
Drawing no.8
You could also start with the line. Try to see what expression you could achieve with different wavy lines or jagged lines.
Try to interweave those lines in complicated ways.
Sophie Taeuber-Arp

If you have overlaid zigzag lines you can create a structure that can be seen as a method called Tiling. It can become ‘Triangular Tiling’, namely Equilateral Triangles (each side the same), Isosceles Triangles (two sides the same) and Scalene Triangles (each side a different length).
A tile is essentially a black cell; a polygon if repeated fits together. It is a key method of making repeat pattern. If you leave it black it becomes a line structure or you can add colour.
Likewise, with wavy lines one can play with a Heptagon, Nonagon and Decagon Tiles. By overlaid zigzag lines or wavy lines you can very quickly make surprising and complex patterns.
The pedagogic layout of the instructions is something I keep coming back to.
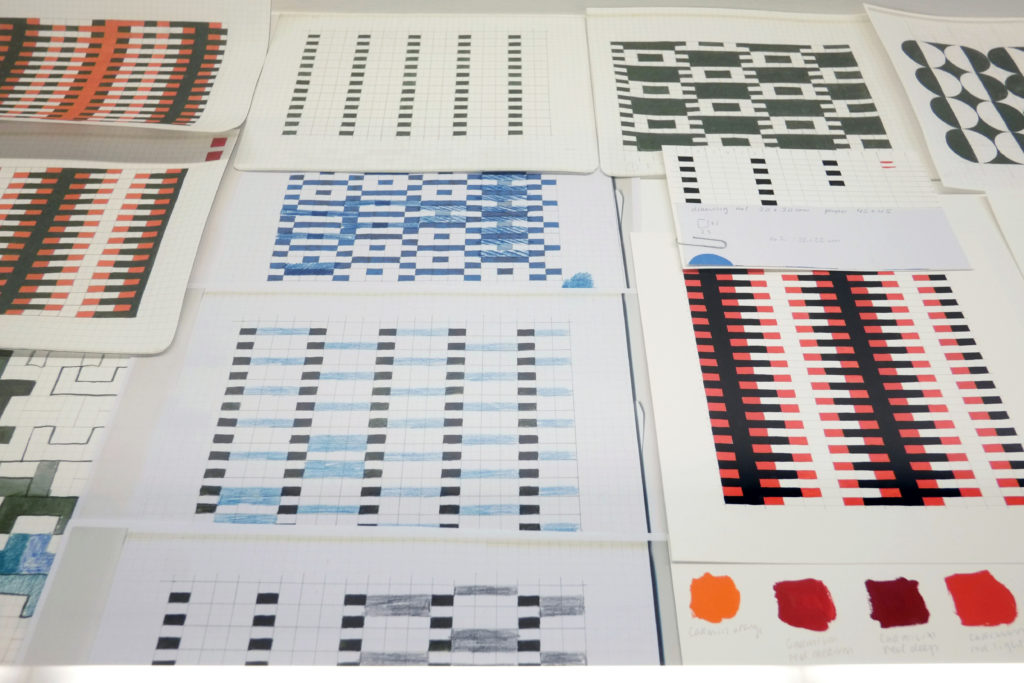
The use of ‘natural’ in the first instruction is fundamental, as one has to take a stand and explore what is most natural for one’s self. It gives you focus and clarity. It is interesting to see in the instructions a parallel to Rudolf von Laban’s teaching of the Five Crystals[7] and to envisage them as a precursor to work by conceptual artists such as Sol LeWitt.
The combination of guidance and room to move, to play and to follow your intuition make Sophie Taeuber-Arp’s instructions a unique and inspiring engagement with pattern, so precise in its aim. If the practitioner works through all the instructions you have basically touched on all the fundamental key elements of designing a pattern.
Nearly one hundred years on, they still hold up in all their clarity.
Endnotes
[1] Please see Medea Hoch essay ‘Sophie Taeuber-Arp’s Interdisciplinary Works within Art History’s System of Categorization, Sophie Taeuber-Arp, Today is Tomorrow, Scheidegger & Spiess, 2014, pp. 213 – 216
[2] Composition vertical-horizontale, 1916, Embroidery, wool, 50×38.5cm. Fondazione Marguerite Arp-Hagenbach, Locarno.
For a reproduction of the work please see ‘The Modernist Textile, Europe and America 1890-1940’, Virginia Gardner Troy, Lund Humphries, 2006, p. 74
[3] Please see Medea Hoch essay ‘Sophie Taeuber-Arp’s Interdisciplinary Works within Art History’s System of Categorization, Sophie Taeuber-Arp, Today is Tomorrow, Scheidegger & Spiess, 2014, p.215
[4] Please see essay on Sophie Taeuber-Arp’s education ‘On Sophie Taeuber-Arp’s Artistic Career and Understanding of Herself: A first Reading of her letters from the 1910s by Sigrid Schade / Walburga Krupp / Medea Hoch, Sophie Taeuber-Arp, Today is Tomorrow, Scheidegger & Spiess, 2014, pp. 259-266.
[5] Correspondence Magazine of Swiss Association of Female Trade and Home Economics Teachers.
[6] ‘>>Try, try…!<<, Variations of a >true artist<’ by Walburga Krupp, Sophie Taeuber-Arp, Variations, Arbeiten auf Papier, Kehrer, 2003
[7] Rudolf von Laban teaching of the Five Crystals uses the cube first (equated with earth and stability) and then circles the cube before introducing the Tetrahedron. See ‘Using the Five Crystals’ ‘Laban for All’, Jean Newlove and John Dalby, Routledge, 2004, pp. 27 – 61


